Explain why it is important to know what the magnitude (brightness) of a star means when having a night sky observation?
The magnitude refers to the brightness of a celestial object where the lower the number the brighter the night sky object. To understand why Astronomers had to go negative with real bright objects, one has to hear this story:
The Story of Magnitude:
A Greek by the name of Hipparchus (remember the Hippocratic Oath), in about 120 BC, was the first to rank stars from the 1st to the 6th magnitude. Hipparchus said all the brightest objects were ranked 1 (second to none) and his 6th magnitude being at the limit of the naked-eye visibility in a dark sky with no light pollution (at the time, fires and/or the moon and/or northern lights, washing out the view of stars).
In the 19th century, photometers made it possible to measure the brightness of dark sky objects much more accurately, so Norman Pogson (1829-1891), in 1856, chose to keep the dimmest objects visible to the naked-eye the same as Hipparchus had them BUT his new scale (known as the Pogson scale) had the difference of 5 magnitudes correspond to a ratio of apparent brightness of 100. Example: That means a magnitude of 1.00 is 100 times brighter than a magnitude of 6.00.
So, the difference of one magnitude of brightness is the fifth root of 100 (not square root or cube root). If you take 100 to the 1/5th power on your calculator, you get the result, the fifth root of 100 = 2.512. Example: So a star with magnitude 1.0 is a little more than 2 ½ times as bright as a 2.0 magnitude star.
Some examples of common sky object magnitudes are:
Sun (apparent magnitude -26.7)
Full Moon (-12.6)
Venus (at it’s brightest, -4.7; because Venus always has thick clouds of sulfuric acid that is very reflective)
Sirius (-1.44)
Arcturus (-0.05) (remember to follow the arc of the handle of the Big Dipper with the saying being: “Arc to Arcturus”, the weapon of the constellation, Bootes, where he and his two dogs (constellation: Canes Venatici) are driving the Big Bear (Ursa Major) around the Pole Star (Polaris, in the Little Bear or Ursa Minor)
Cool fact magnitude story that can help you: The stars that make up the bowl of the little dipper are each about one magnitude apart. Find the north star, Polaris, (which is at the end of the handle of the little dipper) and then look for the two brightest stars nearest Polaris. The brighter little dipper bowl star will be Kochab (means the brighter of the two) and is the beta star of Ursa Minor at a magnitude of 2.07 (Polaris is the alpha star at a magnitude of 1.97) (Note also: Polaris is the 50th brightest star in the night sky) Then the dimmer star next to Kochab is Pherkad (pronounced fair cad; means the fairer or dimmer of the two) and has an apparent magnitude of 3.00). Then the star where the handle of the little dipper meets its bowl is only called, the Greek letter xi, Ursa Minor, has an apparent magnitude of 4.29, around 4.0. And, finally, the star kiddy-corner from the bright star Kochab in the bowl, is the Greek letter nu, Ursa Minor, has an apparent magnitude of 4.95 (about 5.0 so it will be the hardest of the four to make out in the bowl of the little dipper.) So, you have it that in the bowl of the little dipper the stars are almost each one magnitude apart with the brightest being close to magnitude 2.0, the next brightest exactly 3.0 magnitude, where the handle meets the bowl, about 4.0 and the dimmest about 5.0, which if you have any light pollution, you cannot make it out usually.
Final note about apparent magnitudes that is helpful:
The average binocular can see around a 9th magnitude object.
Telescope sizes:
Inches mm faintest magnitude
2 51 10.3
3 76 11.2
4 102 11.8
6 152 12.7
8 203 13.3
10 254 13.8
12.5 318 14.3
So, the Headwaters Telescope a 70 mm primary mirror so can see about 11th magnitude objects at it’s best and my new telescope has a primary mirror diameter of 300 mm so can see about to the 14th magnitude object at its best. This means, 3 magnitudes dimmer or 2.512 to the 3rd power (which is about 15.9 times dimmer object my new telescope can see than the HW telescope can see). A bigger mirror is better.
Note this table:
Magnitude of star difference How many times brighter or dimmer
1 2.512
2 6.31
3 15.9
4 39.8
5 100.0
So, a star magnitude 2.0 is 2.512 brighter than a 3.0 magnitude star and 6.31 brighter than a 4.0 magnitude star and 15.9 brighter than a 5.0 magnitude star and 39.8 times brighter than a 6.0 magnitude star and, finally, 100 times brighter than a 7.0 magnitude star.
Note: When looking at the magnitudes of galaxies or comets then, I don’t even attempt to see magnitudes dimmer (or higher # than) a 12.0 magnitude usually as we don’t really have that dark of skies in our subdivision with Dripping Springs light pollution to the west and Austin light pollution to the east and Bee Cave light pollution to the north but if we took the telescope south west of our subdivision about 20 miles, we could look for the 14th magnitude objects that are over 6 times dimmer than we can see in Headwaters.
The Story of Magnitude:
A Greek by the name of Hipparchus (remember the Hippocratic Oath), in about 120 BC, was the first to rank stars from the 1st to the 6th magnitude. Hipparchus said all the brightest objects were ranked 1 (second to none) and his 6th magnitude being at the limit of the naked-eye visibility in a dark sky with no light pollution (at the time, fires and/or the moon and/or northern lights, washing out the view of stars).
In the 19th century, photometers made it possible to measure the brightness of dark sky objects much more accurately, so Norman Pogson (1829-1891), in 1856, chose to keep the dimmest objects visible to the naked-eye the same as Hipparchus had them BUT his new scale (known as the Pogson scale) had the difference of 5 magnitudes correspond to a ratio of apparent brightness of 100. Example: That means a magnitude of 1.00 is 100 times brighter than a magnitude of 6.00.
So, the difference of one magnitude of brightness is the fifth root of 100 (not square root or cube root). If you take 100 to the 1/5th power on your calculator, you get the result, the fifth root of 100 = 2.512. Example: So a star with magnitude 1.0 is a little more than 2 ½ times as bright as a 2.0 magnitude star.
Some examples of common sky object magnitudes are:
Sun (apparent magnitude -26.7)
Full Moon (-12.6)
Venus (at it’s brightest, -4.7; because Venus always has thick clouds of sulfuric acid that is very reflective)
Sirius (-1.44)
Arcturus (-0.05) (remember to follow the arc of the handle of the Big Dipper with the saying being: “Arc to Arcturus”, the weapon of the constellation, Bootes, where he and his two dogs (constellation: Canes Venatici) are driving the Big Bear (Ursa Major) around the Pole Star (Polaris, in the Little Bear or Ursa Minor)
Cool fact magnitude story that can help you: The stars that make up the bowl of the little dipper are each about one magnitude apart. Find the north star, Polaris, (which is at the end of the handle of the little dipper) and then look for the two brightest stars nearest Polaris. The brighter little dipper bowl star will be Kochab (means the brighter of the two) and is the beta star of Ursa Minor at a magnitude of 2.07 (Polaris is the alpha star at a magnitude of 1.97) (Note also: Polaris is the 50th brightest star in the night sky) Then the dimmer star next to Kochab is Pherkad (pronounced fair cad; means the fairer or dimmer of the two) and has an apparent magnitude of 3.00). Then the star where the handle of the little dipper meets its bowl is only called, the Greek letter xi, Ursa Minor, has an apparent magnitude of 4.29, around 4.0. And, finally, the star kiddy-corner from the bright star Kochab in the bowl, is the Greek letter nu, Ursa Minor, has an apparent magnitude of 4.95 (about 5.0 so it will be the hardest of the four to make out in the bowl of the little dipper.) So, you have it that in the bowl of the little dipper the stars are almost each one magnitude apart with the brightest being close to magnitude 2.0, the next brightest exactly 3.0 magnitude, where the handle meets the bowl, about 4.0 and the dimmest about 5.0, which if you have any light pollution, you cannot make it out usually.
Final note about apparent magnitudes that is helpful:
The average binocular can see around a 9th magnitude object.
Telescope sizes:
Inches mm faintest magnitude
2 51 10.3
3 76 11.2
4 102 11.8
6 152 12.7
8 203 13.3
10 254 13.8
12.5 318 14.3
So, the Headwaters Telescope a 70 mm primary mirror so can see about 11th magnitude objects at it’s best and my new telescope has a primary mirror diameter of 300 mm so can see about to the 14th magnitude object at its best. This means, 3 magnitudes dimmer or 2.512 to the 3rd power (which is about 15.9 times dimmer object my new telescope can see than the HW telescope can see). A bigger mirror is better.
Note this table:
Magnitude of star difference How many times brighter or dimmer
1 2.512
2 6.31
3 15.9
4 39.8
5 100.0
So, a star magnitude 2.0 is 2.512 brighter than a 3.0 magnitude star and 6.31 brighter than a 4.0 magnitude star and 15.9 brighter than a 5.0 magnitude star and 39.8 times brighter than a 6.0 magnitude star and, finally, 100 times brighter than a 7.0 magnitude star.
Note: When looking at the magnitudes of galaxies or comets then, I don’t even attempt to see magnitudes dimmer (or higher # than) a 12.0 magnitude usually as we don’t really have that dark of skies in our subdivision with Dripping Springs light pollution to the west and Austin light pollution to the east and Bee Cave light pollution to the north but if we took the telescope south west of our subdivision about 20 miles, we could look for the 14th magnitude objects that are over 6 times dimmer than we can see in Headwaters.
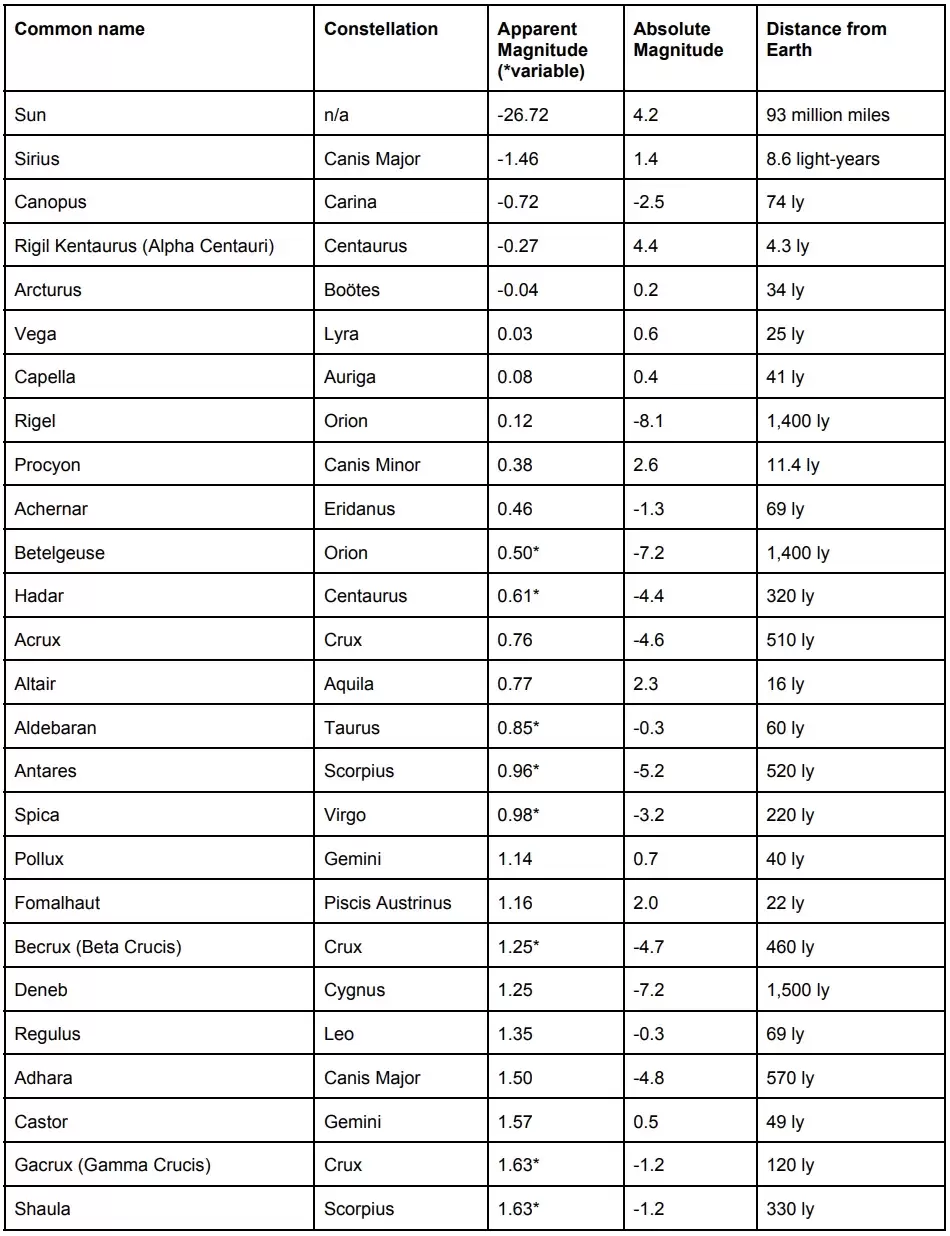
Here is a table absolute and apparent magnitudes of known stars. Look for stars you know.
Noting the table above, I'll pick just one star, Beetlejuice, and compare it to our Sun. If our Sun has an apparent magnitude of -26.72 and an absolute magnitude of 4.2 when 10 parsecs from the Earth AND Beetlejuice has an apparent magnitude of 0.50 but is variable (meaning it changes in brightness over a given period of time) and an absolute magnitude of -7.2. That means that Beetlejuice has an absolute magnitude of 7.2+4.2 = 11.4 more than our Sun. A magnitude of 5 times brighter (meaning 4.2 - 5 = -0.8 is 100 times brighter. A magnitude of 10 times brighter (meaning 4.2 - 10 = -5.8 is 100 times 100 or 10,000 times brighter and Beetlejuice is 11.4 times brighter than our Sun so if Beetlejuice was placed where our Sun is, Beetlejuice would be well over 10,000 times brighter than our Sun as would Rigel in Orion.
Also, if Beetlejuice was placed where our Sun is, Mercury, Venus, Earth, and "yes" even Mars would be inside Beetlejuice thus not exists and Beetlejuice is not the largest star known. There exists ten known stars, so large, that if placed where our Sun is in our Solar System, Jupiter would extend beyond Jupiter's orbit.
Also, if Beetlejuice was placed where our Sun is, Mercury, Venus, Earth, and "yes" even Mars would be inside Beetlejuice thus not exists and Beetlejuice is not the largest star known. There exists ten known stars, so large, that if placed where our Sun is in our Solar System, Jupiter would extend beyond Jupiter's orbit.